publications

2026

1. 

   Local autonomous inference machines for quantum LDPC codes

   Siddhant Midha, and Dmitry A. Abanin

   2026

   Abs Link

   We introduce a local and distributed framework for decoding quantum LDPC codes. Built atop belief propagation (BP), the architecture combines strictly local processing of syndrome information, local feedback, autonomous operation, and highly parallelized decoding. We establish the framework at two complementary levels. First, we show that any code exhibiting a threshold under iterative BP decoding can be promoted to an autonomous local measurement-and-feedback dynamics while preserving that threshold. We then turn to codes for which naive BP does not itself exhibit threshold behavior. Our key observation is that BP can nevertheless provide sufficiently accurate local marginal information: active syndrome defects use these local beliefs to move, pair, and annihilate through asynchronous feedback. We demonstrate numerically that this construction exhibits threshold behavior in the point-like sectors of the two- and three-dimensional toric codes, where conventional BP decoding fails. Exploiting the graph-generality of BP, we then study the inference dynamics on the membrane-like sector of the three-dimensional toric code and in a family of bivariate-bicycle quantum LDPC codes.

2. 

   Algorithmic Locality via Provable Convergence in Quantum Tensor Networks

   Siddhant Midha, Yifan F. Zhang, Daniel Malz, Dmitry A. Abanin, and 1 more author

   2026

   Abs Link

   Belief propagation has recently emerged as a powerful framework for evaluating tensor networks in higher dimensions, combining computational efficiency with provable analytical guarantees. In this work, we develop the first end-to-end theory of tensor network belief propagation for a class of projected entangled pair states satisfying \emphstrong injectivity. We show that when the injectivity parameter exceeds a constant threshold, BP fixed points can be found efficiently, and a cluster-corrected BP algorithm computes physical quantities to 1/\mathrmpoly(N) error in \mathrmpoly(N) time for an N qubit system. We identify a striking phenomenon we term \emphalgorithmic locality: local perturbations of the tensor network affect the BP fixed point with an influence decaying rapidly with distance. As a result, updates to the fixed point after a local perturbation can be carried out using only local recomputation. Moreover, through the cluster expansion, this locality extends to observables, implying that local expectation values can be approximated from local data with controlled accuracy. Our results provide the first rigorous guarantee for the effectiveness of tensor-network belief propagation on a wide class of many-body states, bridging a gap between widely used numerical practice and provable algorithmic performance.

3. 

   Sharp Bounds on Ground State Energy of the SYK Model

   Arpon Basu, Pravesh K. Kothari, and Siddhant Midha

   2026

   Abs Link

   We study the Sachdev-Ye-Kitaev (SYK) Hamiltonian H_\operatornameSYK on n Majorana modes with k-body interactions, and prove that \mathbbE\|H_\operatornameSYK\|_\operatornameop = (1 - o(1))⋅\sqrt2n/k for super-constant k≤o(\sqrtn), where the expectation is over the disorder variables in the Hamiltonian. This confirms the predictions due to Garcia-Garcia, Jia and Verbaarschot’18 and answers a question posed in Feng, Tian and Wei’19. Our results extend to the sparse SYK Hamiltonian. As a corollary, we obtain that the dissipative quantum algorithm of Basso, Chen and Dalzell’24 provably computes the ground state energy of the SYK Hamiltonian up to an O(1)-multiplicative factor for all k<\sqrtn/4. Our key technical idea is identifying an explicit, deterministic linear operator \mathsfx such that a fixed quadratic form of \mathsfx^2\ell exactly equals the expected trace moments of the SYK Hamiltonian for every n and k. This linear operator can be naturally viewed as a \emphtwisted model of bosons on the space of hyperedges of a hypergraph. The problem thus reduces to identifying the spectral edge of \mathsfx, which we show is dominated by the spectrum of a natural n \choose k-dimensional matrix from the \emphJohnson scheme and is straightforward to compute using known results. To show that our bound is sharp, we construct a witness state with a large quadratic form on \mathsfx and transform it into a certificate of a lower bound on the largest quadratic form on H_\operatornameSYK.

4. 

   Belief Propagation and Tensor Network Expansions for Many-Body Quantum Systems: Rigorous Results and Fundamental Limits

   Siddhant Midha, Grace M. Sommers, Joseph Tindall, and Dmitry A. Abanin

   2026

   Abs Link

   Belief propagation (BP) provides a scalable heuristic for contracting tensor networks on loopy graphs, but its success in quantum many-body settings has largely rested on empirical evidence. Developing upon a recently introduced cluster-expansion framework for tensor networks, we rigorously study the applicability of BP to many-body quantum systems. For a state represented as a PEPS satisfying a “loop-decay"condition, we prove that BP supplemented by cluster corrections approximates local observables with exponentially small relative error, and we give explicit formulas expressing local expectation values as BP predictions dressed by connected clusters intersecting the observable region. This representation establishes a direct link between cluster corrections and physical correlation functions. As a result, we show that “loop-decay"\emphnecessarily implies exponential decay of connected correlations, yielding sharp, rigorous criteria for when BP can and cannot succeed, and ruling out its validity at critical points. Numerical simulations of the two- and three-dimensional transverse field Ising model at zero and finite temperature confirm our analytical predictions, demonstrating quantitative accuracy deep in gapped phases and systematic failure near criticality.

5. 

   Beyond Belief Propagation: Cluster-Corrected Tensor Network Contraction with Exponential Convergence

   Siddhant Midha, and Yifan F. Zhang

   PRX Quantum, Jul 2026

   Abs Link DOI

   Tensor network contraction on arbitrary graphs is a fundamental computational challenge with applications ranging from quantum simulation to error correction. While belief propagation (BP) provides a powerful approximation algorithm for this task, its accuracy limitations are poorly understood and systematic improvements remain elusive. Here, we develop a rigorous theoretical framework for BP in tensor networks, leveraging insights from statistical mechanics to devise a cluster expansion that systematically improves the BP approximation. We prove that the cluster expansion converges exponentially fast if an object called the loop contribution decays sufficiently fast with the loop size, giving a rigorous error bound on BP. We also provide a simple and efficient algorithm to compute the cluster expansion to arbitrary order. We demonstrate the efficacy of our method on the two-dimensional Ising model, where we find that our method significantly improves upon BP and existing corrective algorithms such as loop series expansion. Our work opens the door to a systematic theory of BP for tensor networks and its applications in decoding classical and quantum error-correcting codes and simulating quantum systems.

2025

1. 

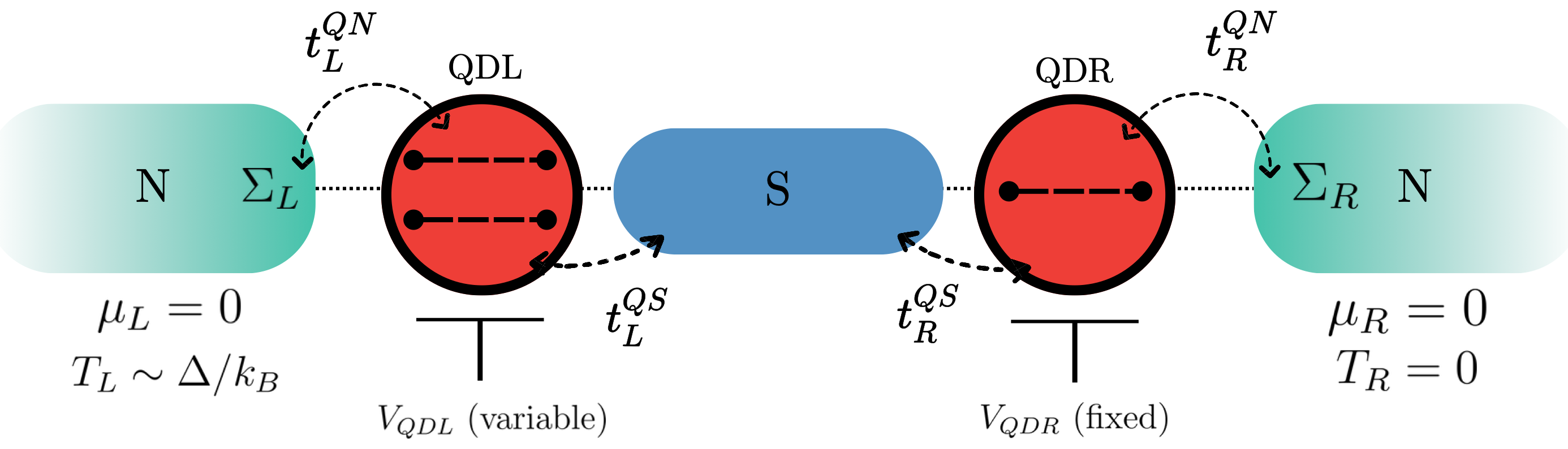   Steady-state dynamics and nonlocal correlations in thermoelectric Cooper pair splitters

   Arnav Arora, Siddhant Midha, Alexander Zyuzin, Pertti Hakonen, and 1 more author

   npj Quantum Information, Mar 2025

   Publisher: Nature Publishing Group

   Abs Link DOI

   Recent experiments on Cooper pair splitters using superconductor-quantum dot hybrids have embarked on creating entanglement in the solid-state, by engineering the sub-gap processes in the superconducting region. Using the thermoelectric Cooper pair splitter setup [Nat. Comm., 12, 21, (2021)] as a prototype, we present a comprehensive analysis of the fundamental components of the observed transport signal, aiming to critically clarify the operating regimes and confirm the nonlocal and nonclassical nature of correlations arising from crossed Andreev processes. By making a nexus with quantum discord, we identify operating points of nonlocal quantum correlations in the CPS device—information that cannot be extracted from the transport signal alone. A notable consequence of our analysis is the finding that contact-induced level broadening of the quantum dot’s discrete energy spectrum, along with its hybridization with the superconducting segment, can lead to shifted resonances in the crossed Andreev process as well as a parity reversal in the thermoelectric current. Our work thereby provides detailed insights into the gate voltage control of the quantum correlations in superconducting-hybrid Cooper pair splitters, revealing new avenues for harnessing quantum correlations in solid-state systems.

2. 

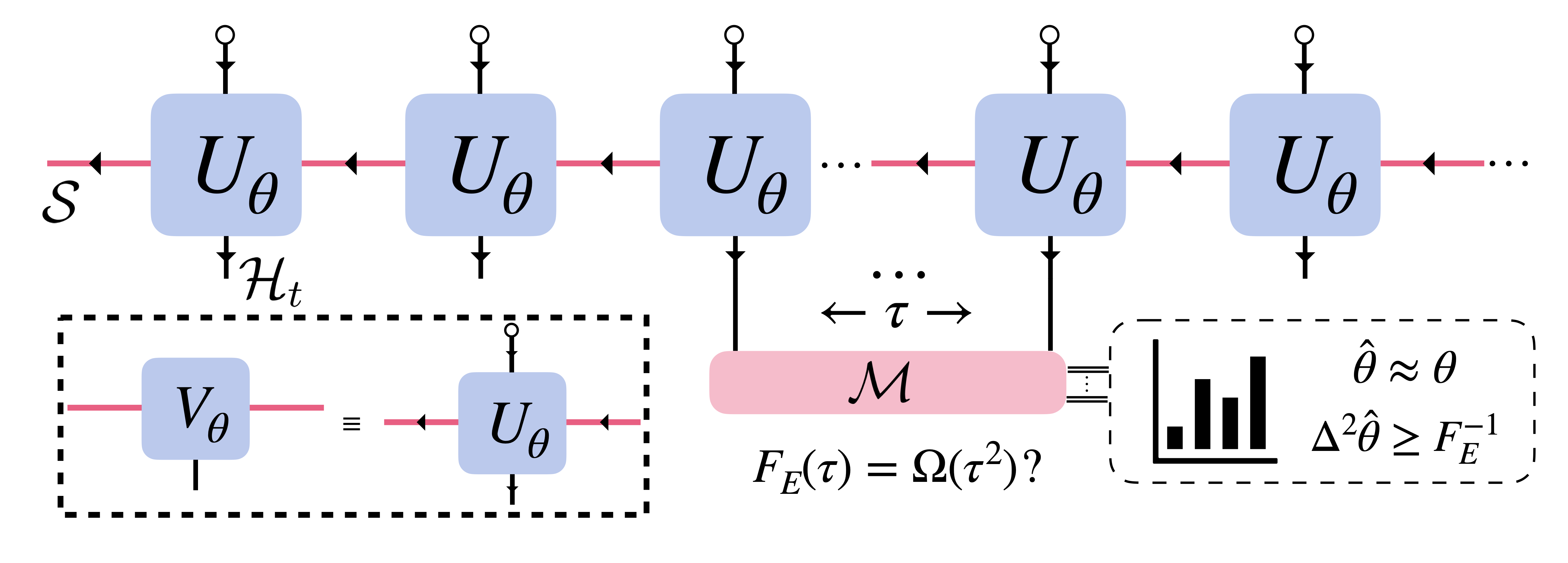   Metrology of open quantum systems from emitted radiation

   Siddhant Midha, and Sarang Gopalakrishnan

   Physical Review A, Dec 2025

   Abs Link DOI

   We explore the task of learning about the dynamics of a Markovian open quantum system by monitoring the information it radiates into its environment. For an open system with Hilbert space dimension D, the quantum state of the emitted radiation can be described as a temporally ordered matrix-product state (MPS). We provide simple analytical expressions for the quantum Fisher information (QFI) of the radiation state, which asymptotically scales linearly with the sensing time unless the open system has multiple steady states. We characterize the crossovers in QFI near dynamical phase transitions, emphasizing the role of temporal correlations in setting the asymptotic rate at which QFI increases. We discuss when optimal sensing is possible with instantaneously measured radiation.

2024

1. 

   Are symmetry protected topological phases immune to dephasing? – a topological electronics perspective

   Siddhant Midha, Koustav Jana, and Bhaskaran Muralidharan

   Journal of Physics D: Applied Physics, Jan 2024

   Publisher: IOP Publishing

   Abs Link DOI

   Harnessing topological phases with their dissipationless edge-channels coupled with the effective engineering of quantum phase transitions is a spinal aspect of topological electronics. The accompanying symmetry protection leads to different kinds of topological edge-channels which include, for instance, the quantum spin Hall (QSH) phase, and the spin quantum anomalous Hall (SQAH) phase. To model realistic devices, it is important to ratify the robustness of the dissipationless edge-channels, which should typically exhibit a perfect quantum of conductance, against various disorder and dephasing. This work is hence devoted to a computational exploration of topological robustness against various forms of dephasing. For this, we employ phenomenological dephasing models under the Keldysh non-equilibrium Green’s function formalism using a model topological device setup on a 2D-Xene platform. Concurrently, we also explicitly add disorder via impurity potentials in the channel and averaging over hundreds of configurations. To describe the extent of robustness, we quantify the decay of the conductance quantum with increasing disorder under different conditions. Our analysis shows that these topological phases are robust to experimentally relevant regimes of momentum dephasing and random disorder potentials. We note that Rashba mixing worsens the performance of the QSH phase and point out a mechanism for the same. Further, we observe that the QSH phase break downs due to spin dephasing, but the SQAH phase remains robust. The SQAH phase shows stark robustness under all the dephasing regimes, and shows promise for realistic device structures for topological electronics applications.

2. 

   Optimized current-density reconstruction from wide-field quantum diamond magnetic field maps

   Siddhant Midha, Madhur Parashar, Anuj Bathla, David A. Broadway, and 2 more authors

   Physical Review Applied, Jul 2024

   Publisher: American Physical Society

   Abs Link DOI

   Quantum diamond microscopy using nitrogen-vacancy (N-V) defects in diamond crystals has enabled the magnetic field imaging of a wide variety of nanoscale current profiles. Intimately linked with the imaging process is the problem of reconstructing the current density, which provides critical insight into the structure under study. This manifests as a nontrivial inverse problem of current reconstruction from noisy data, typically conducted via Fourier-based approaches. Learning algorithms and Bayesian methods have been proposed as novel alternatives for inference-based reconstructions. We study the applicability of Fourier-based and Bayesian methods for reconstructing two-dimensional current density maps from magnetic field images obtained from N-V imaging. We discuss extensive numerical simulations to elucidate the performance of the reconstruction algorithms in various parameter regimes, and further validate our analysis by performing reconstructions on experimental data. Finally, we examine parameter regimes that favor specific reconstruction algorithms and provide an empirical approach for selecting regularization in Bayesian methods.

3. 

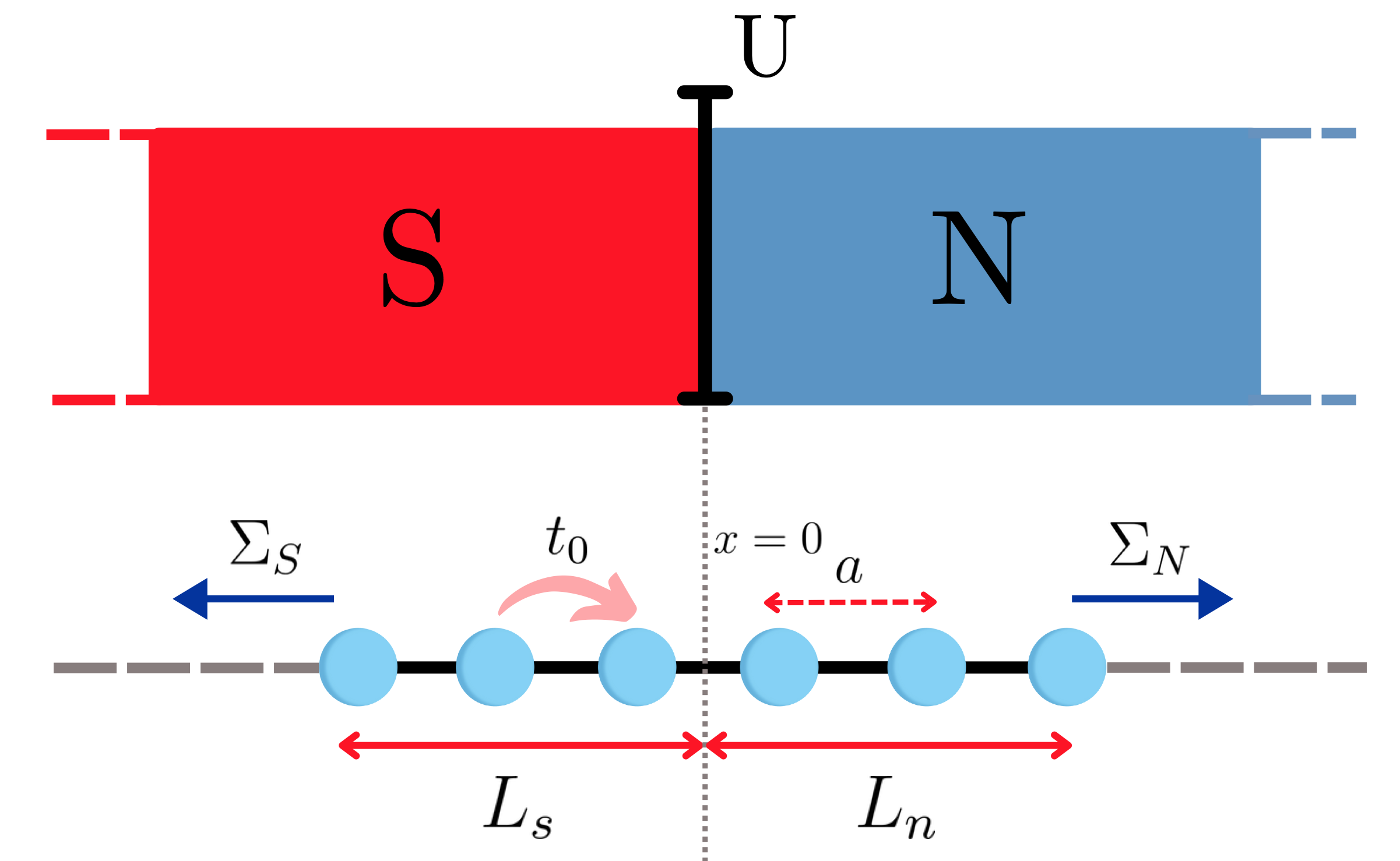   On the microscopics of proximity effects in one-dimensional superconducting hybrid systems

   Siddhant Midha, Roshni Singh, Kaveh Gharavi, Jonathan Baugh, and 1 more author

   Dec 2024

   arXiv:2411.12733 [cond-mat]

   Abs Link DOI

   Investigating the microscopic details of the proximity effect is crucial for both key experimental applications and fundamental inquiries into nanoscale devices featuring superconducting elements. In this work, we develop a framework motivated by experiments to study induced superconducting correlations in hybrid nanoscale devices featuring layered superconductor-normal heterostructures using the Keldysh non-equilibrium Green’s functions. Following a detailed method for analyzing the induced pair amplitude in a prototypical one-dimensional hybrid, we provide insights into the proximity effect within and outside the Andreev approximation. Our analysis also uncovers a disorder-induced crossover in the correlation patterns of the system. By elucidating the spectral distribution of the induced pair amplitude, we investigate the pair correlations established in a recent experiment [Phys.Rev.Lett.128,127701], providing a theoretical basis for the enhanced Cooper pair injection demonstrated through the lens of the induced pair correlations, thereby establishing the promise of our methods in guiding new experiments in hybrid quantum devices.